Hollow Shaft Loaded with Sinusoidal Torque on Free End

Let us consider longitudinal vibrations of a steel shaft. Its left end is fixed. A torsional couple of forces is applied to the right end starting from time t = 0. The forces torque changes under the law M=M0∙sin(ωt).

It is necessary to find the maximum torsion angle and the maximum shearing stresses in the shaft.

Shaft length l=1 m. Cross-section is annular with inner diameter d1=74 mm and outer diameter d2= 80 mm with thickness of 3 mm. Modulus of elasticity E=200 GPa, Poisson ratio ν=0.29, density ρ=7900 kg/m3. Amplitude and frequency of external torque are correspondingly equal P0=1 kNm and ν0=ω/2π=500 Hz.

Studies of longitudinal vibrations and torsion oscillations of beams are mathematically similar. That’s why we can use the solution of longitudinal vibrations of the beam to the left end of which the force changing under the law P=P0∙sin(ωt) is applied to the torsion angle j finding:

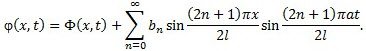
where

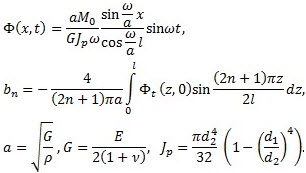
The maximal shearing stress in the section (appears on the outer circumference) is calculated using the formula:

It is positive if its torque is directed counter-clockwise from the outer normal line side.

Limited by the 31 terms of series (n=0…30), we create a graph of angles of rotation of sections with coordinates x=l (right end of the shaft), x=0.75l, x=0.5l и x=0.25l according to the time t on ten intervals of outer torque.

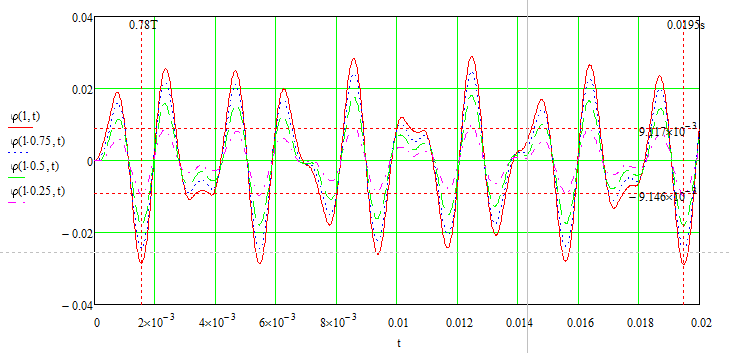
Graphs of angles of rotation of four sections according to the time

The maximal angle of rotation is on the right end of the shaft and is 9.146E-003 rad.

Let us create graphs of maximal shearing stresses in the section for three sections with coordinates: x=0 (closing), x=0.5l and x=l (right end of the shaft) according to the t time on ten intervals of outer torque.

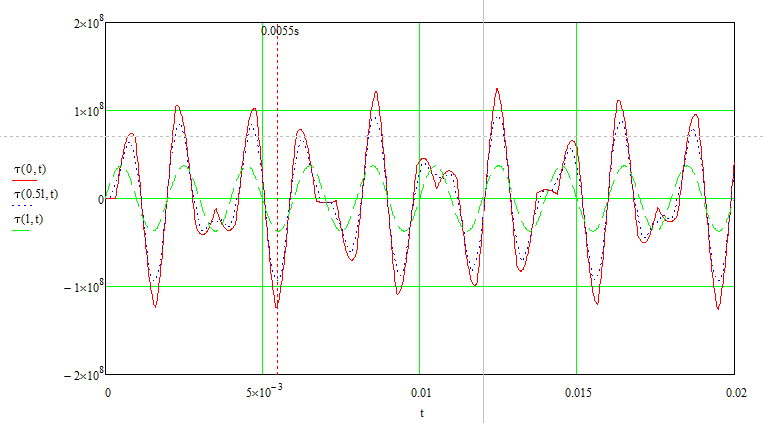
Graphs of maximal shearing stresses in the section according to the time.

Let us calculate AutoFEM Analysis study: We create two studies, one Transitional processes, another Mode superposition with the same loads and restraints.

We create a full restraint on the left butt end of the shaft. The right end will stay free. To exclude the shaft bending we set kinematic restrictions on the radial displacements (radial restrictions are equal to zero) for the outer and inner cylindrical surfaces- for that purpose we apply partial restraints in the cylindrical coordinate system. Displacements by radius and axis are restricted, displacements around the circumference are permitted, Z axis of the cylindrical coordinate system is directed along the shaft axis. On the free end we apply torque load, the value of the load is specified using graph:

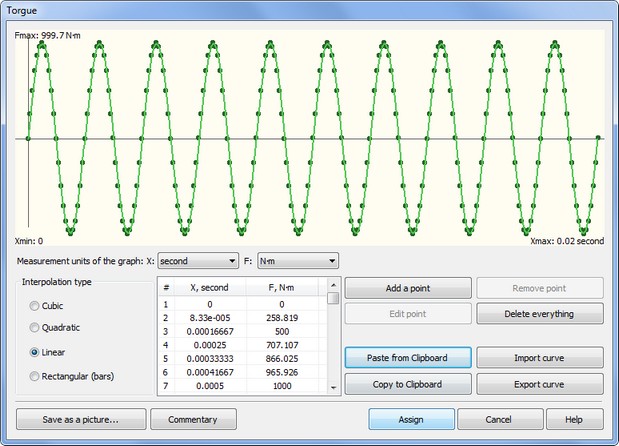
For both of the studies, we set the finite modeling time 0.02 s, the time step of integration 1E-005 s. The method of time integration: Newmark. We set a number of the lower natural frequencies in the Mode superposition study: 5.

The angle of rotation of the section on the free end of the shaft is calculated by the formula φ=2uyB/d2, where uyB - projection of point B displacement on the 0 y-axis (found from the calculation in AutoFEM Analysis).

On the figure below, you may see the dependencies graphs of free shaft end angle of rotation from time for an analytical solution, a numerical solution using Transitional process and Mode superposition.

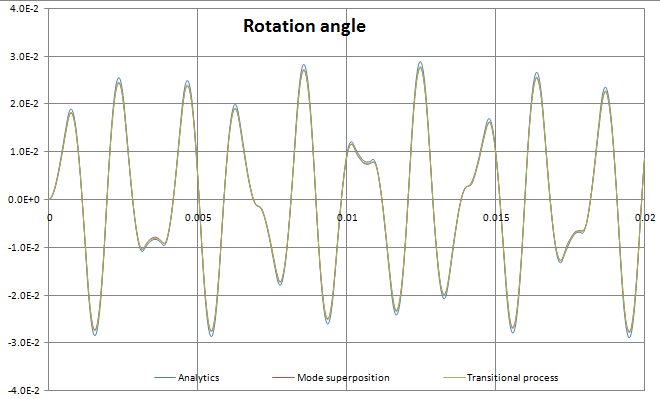
Graphs of rotation angle of free shaft end using analytical solution and solutions by two AutoFEM methods

On the figure below, the difference between analytical solution and solutions by two AutoFEM Analysis methods are shown.

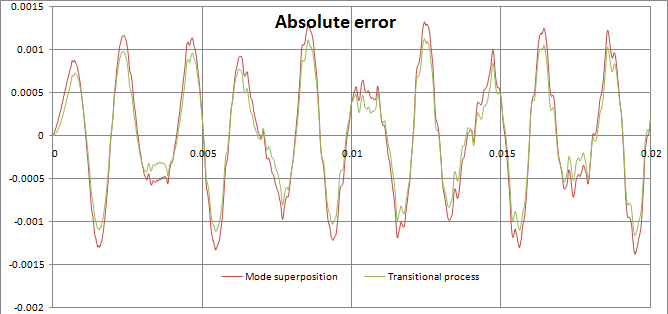
Graphs of differences of rotation angle of shaft end using analytical solution and solutions by two AutoFEM methods

 

On the figure below, the graphs of shearing stress τxy in the point A using analytical solution and numerical solutions by two methods in AutoFEM Analysis are shown. Positive directions of the shearing stress of analytical solution τA and considered in the theory of elasticity τxyA (by AutoFEM Analysis) are shown on the figure “ The finite element model with applied loads and restraints”.

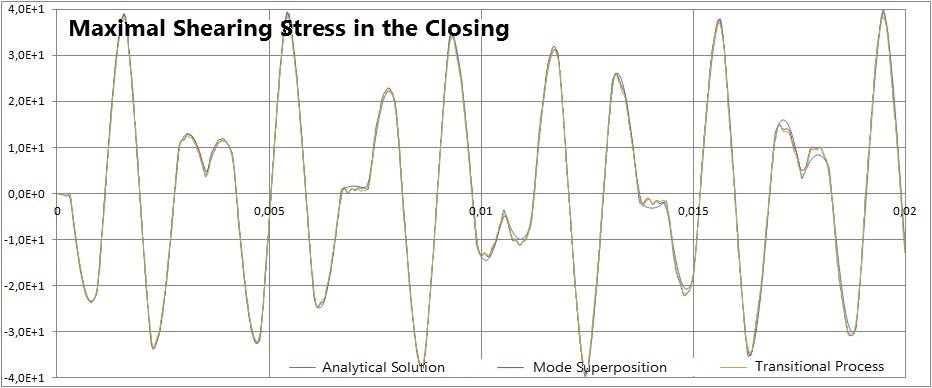
The graphs of shearing stress in the point A using analytical solution and two numerical solutions by two methods in AutoFEM Analysis are shown.

 

On the figure below, the graphs of shearing stress differences τxy in the point A using analytical solution and numerical solutions by two methods in AutoFEM Analysis are shown.

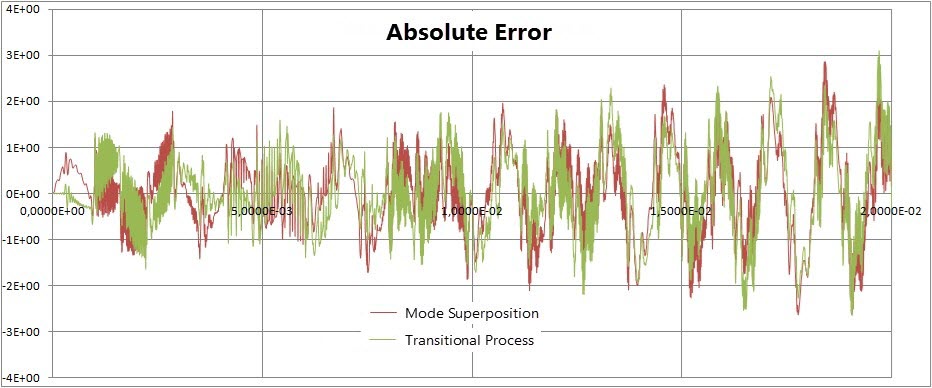
The graphs of shearing stress differences in the point A using analytical solution and two numerical solutions by two methods in AutoFEM Analysis are shown.

 

After carrying out calculation with the help of AutoFEM, the following results are obtained:

Table 1.Parameters of the finite element mesh

Table 2.Parameters of  temporal discretization

Table 3. Transitional processes, maximal torsion angle*

Table 4. Mode superposition, maximal torsion angle*

Table 5. Transitional processes, maximal shearing stress*

Table 6. Mode superposition, maximal shearing stress*

 

Conclusions:

The maximal angle of rotation of shaft free end calculated using AutoFEM Analysis is: for the Transitional process 2.7775E-02 rad (relative error 4.00%), for the Mode superposition 2.7575E-02 rad (relative error 4.69%). The maximum shearing stress at the A point found using AutoFEM Analysis is: for the Transitional process 38.434 MPa (relative error 3.68%), for the Mode superposition 39.210 MPa (relative error 1.73%).

*The results of numerical tests depend on the finite element mesh and may differ slightly from those given in the table.

 

Read more about AutoFEM Static Analysis

autofem.com

Return to contents