Distributed Heat Source applied to the Cylindrical Surface inside the Disk

Let us consider a problem of steady-state distribution of the temperature at the end-face of the disk inside of which there is a heater in the form of a cylindrical surface with a radius rd=20 mm, power P=100 W, and on the periphery a constant temperature of 273.15 oK is held.

Parameters of a disk: metal disk of thickness d=5 mm, radius  R=100 mm and thermal conductivity K=50 W/(m • oK) – thermal conductivity of a disk inside and outside the cylindrical surface of the heater is the same. [Alloy Steel (SS)].

(see figure).

Solution of this problem, in which the source is considered as distributed, can be obtained by solving the problem for a point heat source (as in the previous example). Let the origin coincide with a center of a circular surface of a disk. The differential equation that is to be solved for a point source has the form:

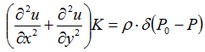
where ρ – density of distributed power. Solution of this equation is known. For our case: ρ = P/ (2π•rd•d) = P/S, where S – area of cylinder surface. By taking into account distribution of the power around the ring of the cylinder, let us express the temperature as a total power of all applied point sources by integrating the delta-function, on the right-hand side, over the point P0 . As a result, we obtain an equation:

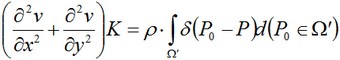
where Ω – is a set of points on the surface of a disk, Ω’ – is a set of points that lie inside the surface of a disk and bounded by a ring of a cylinder on which the power is prescribed. Solution of this equation ν is a linear combination (integral) of solutions for point sources:

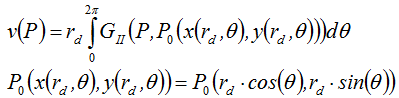
where GII – is a Green’s function for a power defined by the formula ρ = P/S, for distribution on the surface. After integrating, we obtain:

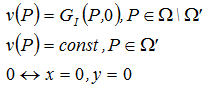
Here GI – is a Green’s function for a power defined by the formula  ρ = P/S for a point source distributed on the edge. This implies that the magnitude of the temperature ν will coincide with the temperature from a point source (located at the center of a circle) for points that lie outside with respect to the ring on which the distributed heat source is prescribed. In internal points, the temperature will take the value equal to some constant. Note that ν – is a continuous function and that is why the value of the constant inside the embedded cylinder is always known.

Let us compare analytical solution with the solution obtained from AutoFEM. After carrying out calculation the following results are obtained:

Table 1. Parameters of finite element mesh

Table 2. Result "Temperature"

 

Compare this plot with a field generated by the source distributed along the edge. We see that they coincide (the plot is made based on calculations with a quadratic element).

Conclusions:

For a given problem we obtained a realistic picture of the temperature field. The relative error of the numerical solution compared with the analytical solution not exceed 0.03% when using linear element on the edge of a disk.

In addition, we note that modeling of a problem with distributed source by a point source was quite possible. We do not claim that it is always possible. However, for certain geometries this can be done. For example, in our case, the distributed heat source had a central symmetry.

 

*The results of numerical tests depend on the finite element mesh and may differ slightly from those given in the table.

 

Read more about AutoFEM Thermal Analysis

autofem.com

Return to contents