Large Deflection of a Circular Plate (surface)

Large Deflection of a Circular Plate Under a Uniformly Distributed Load

Let us consider a circular plate with the radius a and the thickness h. The plate is clamped and subjected to a uniformly distributed load with the intensity q (see figure).

The finite element model of a clamped circular plate under a uniformly distributed load

The finite element model of a clamped circular plate under a uniformly distributed load (large deflection)

Let us use the following initial data: the radius of the plate a is 0.25 m, the thickness of the plate h is 0.005 m, the load intensity q is 1E+05 Pa.
Material properties are E=2.1E+011 and ν= 0.28.

Let us use the following approximate formula to calculate displacements of the plate center:
,
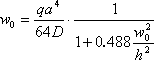where

is the bending stiffness of the plate.

Solving this equation for w0, we obtain the value of the maximum deflection, which is expected in the center of the plate: w0= 2.3258E-003 m.

After carrying out calculations (taking into account nonlinearity) by the AutoFEM Analysis the following results are obtained (number of load steps is 6):

Table 1.Parameters of the finite element mesh

Finite Element Type	Number of Nodes	Number of Finite Elements
Linear triangle	921	1712
Quadratic triangle	3553	1712

Table 2.The result “Displacement, magnitude”

Numerical solution Displacement w0*, mm	Analytical solution Displacement w0, mm	Error δ =100%\|w0- w0\|/\| w0 \|
2.3059	2.3258	0.86
2.3042	2.3258	0.92

Large Deflection of a Circular Plate Under a Uniformly Distributed Load, the result “Displacement, magnitude”

Conclusions:

The relative error of the numerical solution compared to the analytical solution is equal to 0.92% for quadratic finite elements.

*The results of numerical tests depend on the finite element mesh and may differ slightly from those given in the tables.