 |
 |
 |
 |
 |
 |
AutoFEM Analysis Vibrations of a Simply Supported Plate Because of the Middle Point Oscillation |  |
|
|
|
|
|
|
AutoFEM Analysis Vibrations of a Simply Supported Plate Because of the Middle Point Oscillation | |
Vibrations of a Simply Supported Plate Because of the Middle Point Oscillation
Let us consider a simply supported plate, the middle section of which harmonically oscillates with an amplitude of 0.5 mm. (see figure)
|
Our aim is to find oscillation amplitudes of a point with coordinate x using the specified frequencies.
Let us use the following initial data: length of the plate L = 850 mm, the cross section is a rectangle with width b = 75 mm, height h = 5 mm. Harmonic force is applied at a point with x=0.5L=212.5 mm.
Parameters of the material: modulus of elasticity E=2.1E+011Pa, Poisson's ratio ν=0.28, density γ=7800kg/m3.
Classic analytical solving
Natural (resonant) frequencies of the system are:
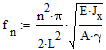
Where Jx=bh3/12 - the moment of inertia of cross-section;
f1,2,3,4= 16.2826; 65.1304; 146.5434; 260.5216. Thus, first natural frequency falls in range 4Hz to 32Hz.
Dynamic deflection at point x is calculated by formula:
.
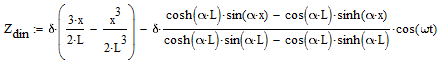
Maximum deformation is attained at ωt=π. Deflection under the dynamic load at 4Hz to 32Hz: ΔZdyn = 0.344039; 0.344910; 0.346381; 0.348479; 0.351244; 0.354734; 0.359022; 0.364205 mm.
Numerical solution
Let us solve this study by AutoFEM Analysis package. Both ends are restrained to simulate simple support: displacements of the left end along XYZ-axis are forbidden and rotation only around Y-axis is allowed; displacements of the right end along YZ-axis are forbidden and rotation only around Y-axis is allowed.
|
The finite element model with applied loads and restraints |
First eigenfrequency is equal to f(1)n =16.286 Hz (the result "Mode 01 (16.286 Hz)" of the study "Study 2 (eigenfrequencies)").
Vibrational amplitudes have the following values: Z*dyn= see table 2 (results "4.000 Hz-Form, Amplitude Z ... 32.000 Hz-Form, Amplitude Z" of the study "Study 3 (forced oscillations)").
Let us compare the results of calculation:
Table 1. Parameters of the finite element mesh
Finite element type |
Number of nodes |
Number of finite elements |
linear triangle |
585 |
352 |
Table 2. The results
Frequency ff , Hz |
Analytical solution |
Numerical solution |
Error δ = 100* | R* - R | / | R |, % |
4 |
0.344039 |
0.3460 |
0.57 |
8 |
0.344910 |
0.3478 |
0.84 |
12 |
0.346381 |
0.3507 |
1.25 |
16 |
0.348479 |
0.3548 |
1.81 |
20 |
0.351244 |
0.3603 |
2.58 |
24 |
0.354734 |
0.3672 |
3.51 |
28 |
0.359022 |
0.3756 |
4.62 |
32 |
0.364205 |
0.3858 |
5.93 |
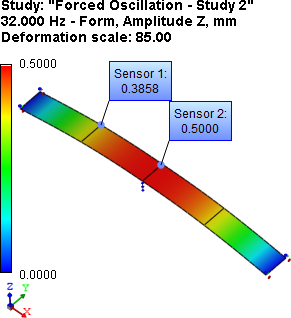
*The results of numerical tests depend on the finite element mesh and may differ slightly from those given in the table.
Read more about AutoFEM Oscillations Analysis
