Superposition of Frequency Modes

In the mode superposition method, the system (1) that consists of N related differential equations of motion is transformed in the equivalent system that consists of n independent ordinary differential equations. For this purpose the natural frequencies and oscillation forms of the system are calculated. Then, using fundamental properties of oscillation forms, the system (1) that consists of many thousands and millions of equations is transformed in the system of independent differential equations of dimension equal to the number of oscillation forms selected for equalization. The resulting solution of the system (1) is presented as the sum of oscillation forms that were used for equalization, with calculated weighting factor. The advantage of the method is its computational efficiency at the dynamic study solution stage, i.e. solving of the reduced equations system is much more effective then solving of full equations system (1). You should take into account that preliminary calculation of natural frequencies and oscillation forms is a resource-demanding task and it should be taken into account when selecting method of dynamic task solution. One more important restriction of the mode superposition is impossibility of its usage for nonlinear studies.

Accuracy of the method depends on the oscillation forms, selected for equalization, and their quantity. Quantity of natural modes necessary for the exact calculation is in the range of 10-15 depending on the complexity of the model and the nature of the modelled impact. The greater the quantity, the more accurate the result, but the calculation time is increased correspondingly.

In general, the Mode Superposition method may be recommended for modelling of long time linear dynamic processes. When the types and quantity of oscillation forms are selected correctly the method provides satisfactory accuracy and efficiency of the solution.

There are two types of mode superposition module in AutoFEM Analysis.

1. Dynamics - Mode Superposition is based on preliminary solved Frequency Analysis study. While creating a such study, the user should select existing frequency analysis study, the modes of which will be used in dynamic analysis solving.

The button to create Mode Superposition study

The dialogue to select an existing frequency study

The selected study is shown in the study tree and can be replaced with the study based on the same set of solids (to preserve the applied boundary conditions).

Basic frequency study

The advantages of the described approach are

•the separateness of frequency study solving from the dynamic analysis. It allows the user to save time during multiple dynamic study solving;

•the possibility to easily change basic frequency study, for example, to verify convergence changing the quantity of modes;

•the easiness of dynamic boundary condition applying;

•no necessity to create finite-element mesh;

•the possibility to control modes taking part in the calculation (can be useful for eliminating degenerated modes).

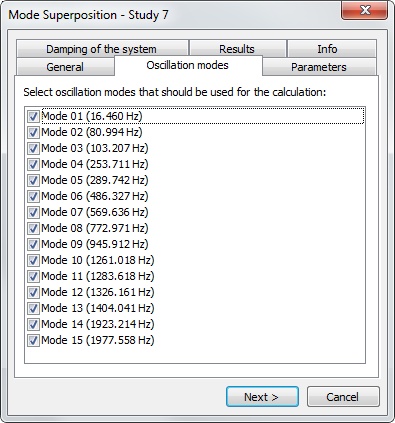
2. Frequency & Mode Superposition. This mode combines solving frequency study and then dynamic study. Before calculation, user should define natural oscillation forms for which the equalization will be performed. It can be done by one of the two ways:

1.On the Solve tab in the "Use frequency analysis results" group select already calculated study of frequency analysis (study type: "Natural frequencies"). All natural modes from the selected study will be used for finding solution of mode superposition.

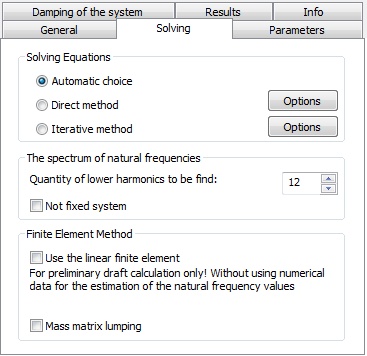
2.Activate natural modes solving mode directly in the dynamic study. You need also select quantity of natural modes. Other control elements mainly coincide with the corresponding parameters of frequency analysis.

Return to contents

autofem.com